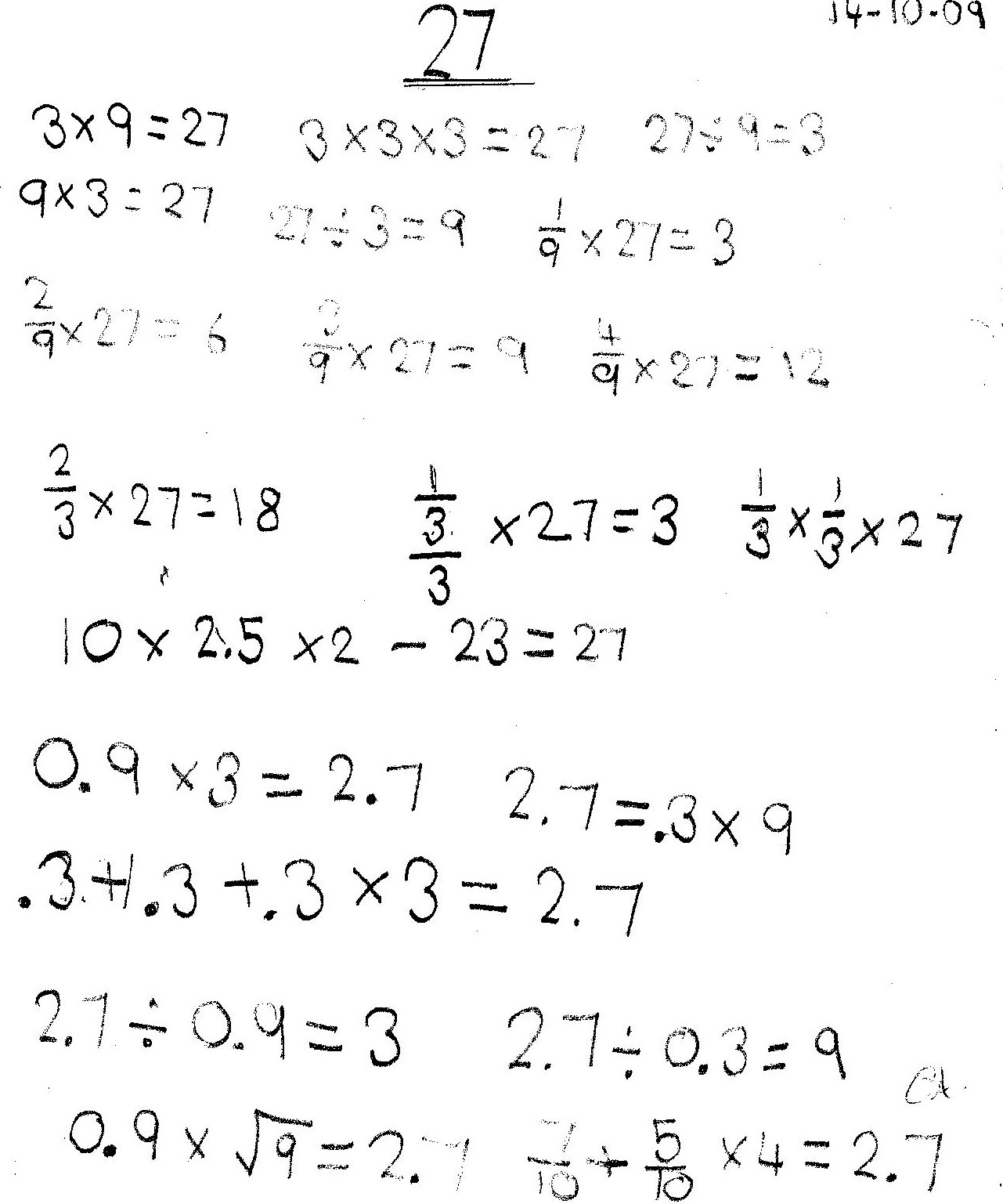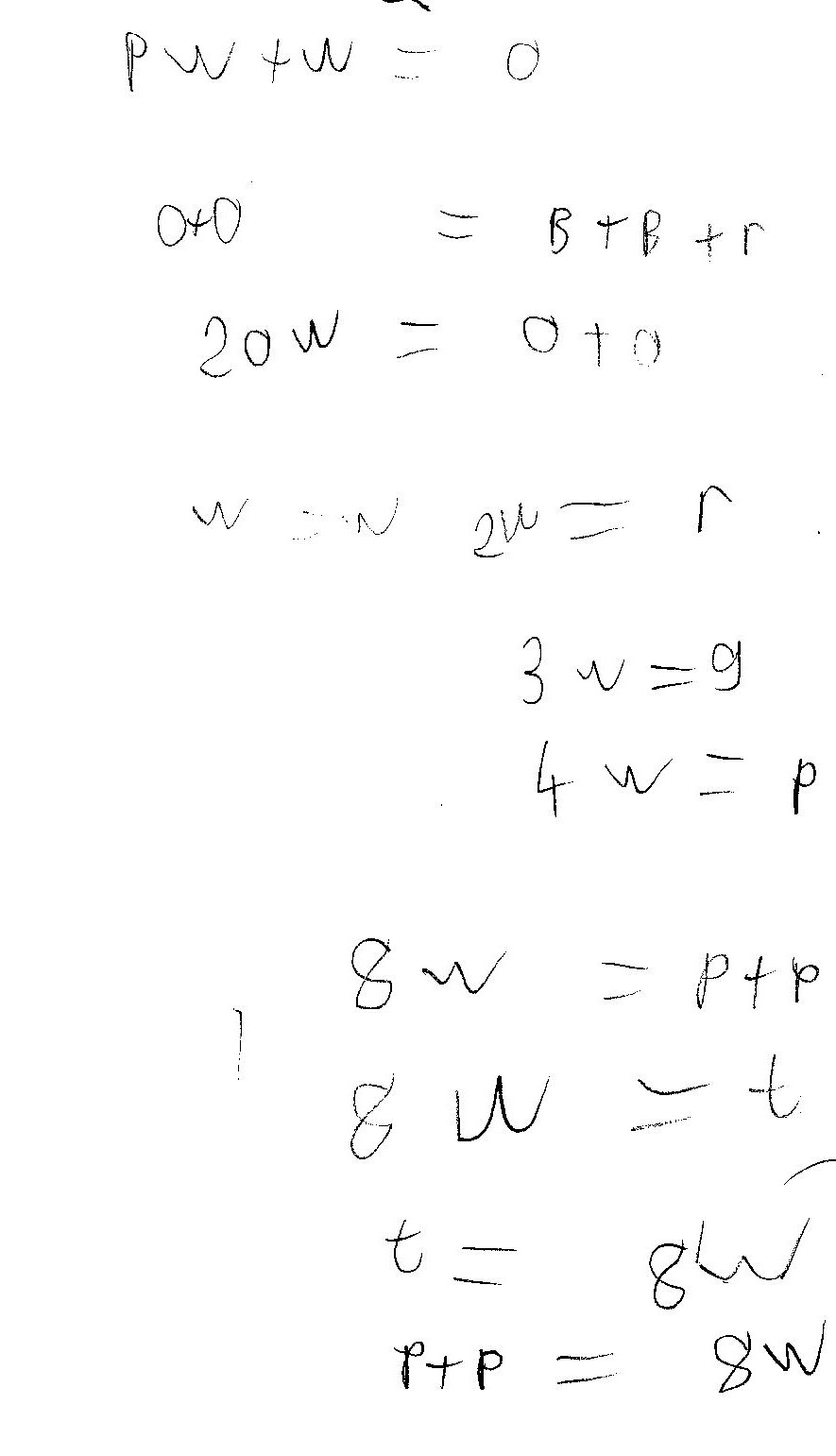2.2.1 Filmed discussion
Here Caroline writes about her work and outlines some of the key ideas from Madeleine Goutard’s writing which have inspired her. This article also includes video extracts from Caroline’s classroom and samples of her children’s written work to illustrate her approach.
Improving mathematics teaching through studying the writing of Caleb Gattegno and Madeleine Goutard
Background
Four years ago (in 2006) I worked through Caleb Gattegno’s ‘Numbers in Colour’ (1957) text books in the hope of improving my own understanding, and therefore my teaching, of mathematics. Written as a dialogue with the learner, Gattegno revealed to me a fascinating subject, very different from the one I was taught at school! Most importantly, everything I learnt I felt I had discovered for myself.
Hoping to give my children the same excitement of discovery and power over the subject, I initially used the text books with a class of year 3 and 4 children. From my very first attempts to teach with Gattegno’s approach, I knew that the children were thinking differently about the subject: they had a new sense of purpose, discovery and power, and were gaining greater flexibility and creativity with calculation. I then came across the work of Madeleine Goutard (in turn influenced by Gattegno) who had interpreted this approach with classes of young children, with astonishing results.
Mathematics and Children, by Madeleine Goutard documents her work advising teachers in Quebec in the 1960s, and teaching mathematics to primary school children. Goutard describes and gives evidence of how the children she taught routinely gained mastery of addition, subtraction, division, multiplication and powers, by the age of 7 or before.
Inspired by seeing examples of her children’s independent mathematical writing, I hoped to understand and perhaps even replicate the fluent, complex and creative mastery of expressions achieved by Goutard’s children. I also wanted to understand the connection between this independent writing and the positive effects of Gattegno’s approach which my children were already experiencing. However, it soon became clear to me that to understand her theory fully and to have any hope of reaching Goutard’s level of achievement as a teacher, I would have to work in such a way that involved a constant reappraisal of my teaching; an opportunity to teach a wider age-range of children than just years 3 and 4, and a careful study of the texts.
I therefore began to teach mathematics in this way to all the children (Reception to Year 6) in my school. Now, three years into my research, encouraged by rising SATs results and children’s confidence, I am convinced that this early mastery is not only possible but vital to children’s later success in, and enjoyment of, mathematics. Whilst I do not claim to have reached Goutard’s level of skill as a teacher, I have seen enough to share her belief (and that of Gattegno) that young children are capable of far more than is currently expected of them.
As a result of this research, I now understand that the key to this theory is to teach children, from their very first encounter with the subject, what Gattegno called the ‘algebra’ of operations, made possible through specific use of Cuisenaire rods. “the rods act as an algebraic model – for the algebra of arithmetic – making it possible to start with algebra instead of counting. It made sense to the students. They paid attention to the perceptible attributes and had very little to memorize. Therefore they could re-invent, easily and on every occasion, what was needed to solve a problem, and did not worry they might forget facts only held in their memory.” p. 3 (Gattegno 1987).
The Cuisenaire rods are used as a way of presenting what can be generalized about an number and number operations. In this way children become aware of addition as commutative and associative, division as repeated subtraction, multiplication as repeated addition, inverse operations, etc. before applying these ‘rules’ to any specific numbers. Importantly, the labeling of an operation with a mathematical sign can be agreed between teacher and children in the context of the shared experience of constructing an arrangement of rods. No further language is require as the arrangement itself and the act of constructing it contain all that is necessary to see what is distinct about an operation and what is shared with other operations. A shift of focus is all that is needed to re-label the same arrangement with a different mathematical sign. By encouraging independent ‘free’ mathematical writing, children then explore the algebra of operations (often as a series of transformations), working on all operations at once, and express their discoveries for themselves. In this way, the Cuisenaire rods can be used in all areas of number work, allowing children to constantly revisit their discoveries and build on previous learning.
What follows is an account of how my classroom-based research and my study of Goutard and Gattegno’s theory of teaching have led me to these conclusions.
Goutard’s theory of teaching mathematics to young children
“It is generally agreed that concrete experience must be the foundation of mathematics learning. When children find it difficult to understand arithmetic it is at once suggested that this is because it is too abstract; for small children the study is then simply reduced to the counting of objects. It seems to me that there has perhaps been too great a tendency to make things concrete and that perhaps the difficulties children experience spring from the fact that they are kept too much at the concrete level and are forced to use too empirical a mode of thought…
“The advantages of a material such as the one proposed by George Cuisenaire is that, paradoxically as it may seem, it enables children to reach an understanding of mathematical structures and frees them from the necessity to have recourse to a concrete support.” p.2 (Goutard 1964,2017).
‘Concrete’ is defined as “existing in material form…, denoting thing as opposed to quality, state or action, not abstract” (Concise Oxford Dictionary p.195, 1982). Although the rods can be seen as concrete as they exist in material form, it seems to me there is a danger in using only their concrete property, particularly with very young children, thereby underestimating children’s potential for abstract thought.
Goutard proposed that young children could move on quickly from the empirical mode of thought to making ‘rational discoveries at the level of structures’ (systematisation) and from there towards mastery of these structures.
I have found the following descriptions helpful in identifying the stages in my children’s work.
Empirical – “based or acting on observation or experiment, not on theory; regarding sense-data as valid information; deriving knowledge from experience alone.” Concise Oxford Dictionary 1982 p.315 “..one starts gleaning facts. This is done by trial and error, the results being accepted or rejected according to the criterion imposed on oneself. These facts are gathered at random, everybody gleaning what he can… Nevertheless they will only have been able to gather material. …The children have acquired more a technique than knowledge founded on reasons” p.6. (Goutard 1964,2017).
For example with respect to addition, children might be finding different trains of rods which are equivalent to another rod.
Systematisation – “.. to organise experience, to clarify facts so as to fill gaps if some are found, to propose groupings of some significance, in a word to invent sure means with which a thorough study of the situation could be undertaken.” p.8 (Goutard 1964,2017),
With addition, children may now be attempting to find out if they have found all the ways of partitioning a rod into smaller rods by grouping them in some way. They may use their knowledge that addition is commutative, or substitute two or more rods for an equivalent length to find new combinations of rods. They may begin to order their partitions according to some rule they have agreed.
Mastery – Goutard describes activities leading to mastery…. ”It is therefore towards a deeper understanding of the structures involved in these situations that the above discoveries take us. Every element or group of elements is seen to potentially contain the infinite set of which it is part, as soon as the dynamic link between the elements has(?) been noticed” p.18 (Goutard 1964,2017).
For example, in addition, children may discover that they can move from any one pair of complements to any other by adding the same amount to one rod as they have subtracted from the other.
These three phases of empiricism, systematisation and mastery are therefore crucial to Goutard’s theory of teaching mathematics and provided the focus for this part of my research. I needed to identify what I believed to be the characteristics of children working at each phase, understand its value, and find ways of moving children through the phases towards mastery.
The Research Process
My research follows a specific cycle:
From reading the texts:
- I identify something I want to understand better or questions I want to answer (in this case understanding Goutard’s 3 phases of children’s thinking);
- I translate this into a classroom activity and video groups of children working on it;
- I watch the clips back and look more closely at children’s responses both planned and unexpected;
- I return to the text with more practical understanding, and so begin the process again.
The aim of these videos and the research project as a whole is to try to show a continuous cycle of classroom research rather than present polished findings or recommend a specific pedagogy. Therefore, in what follows I have included a commentary to accompany each series of video clips showing how and why activities changed.
The videos that follow in Part 1 explore the value of the empirical phase of children’s work. Discover how I tried to avoid them working at ‘too empirical a mode of thought’
Part 1 discussion What is the value of the empirical phase of children’s work and how can I avoid them working at ‘too empirical a mode of thought’?
In this sequence of clips, I use an activity in which I believe children are working empirically. The idea comes from Goutard’s description of how she introduces fractions.
“In introducing fractions, the key to success is variety, change, diversity of points of view, and teaching as little as possible. Ordinarily I give only one fraction to the children, enough to furnish them with a terminology and written style; they discover the rest…We should engage in group discussion where possible answers are examined and the children should conduct experiments to determine if they are acceptable or if they must be modified. Only in this way can we truly learn. When we deny children the right to make mistakes, we do their work for them and tell them what they should be discovering for themselves” p.75 (Goutard 1974)
I want to find out when children are given as little as Goutard suggests, how much will they be able to discover for themselves and whether the act of constructing the rest gives them the creativity and understanding that Goutard’s children showed? I want to discover for myself what the value of the empirical phase is and how will it lead to systematisation?
Class 2 (Year 2 and 3) Video sequence. Term 1 Naming fractions: “How many fit it?”
In each of these clips (Cl2A to D), children are naming rods, taking turns to suggest and justify a name by comparing it with a rod already named. This activity allows me to hear the child’s reasoning and to find out which comparisons they are using.
Figure 2.3: C12A
Clip C12A demonstrates the importance of comparison. When the first child says that the rod is 3, it could just be the third rod set out, when prompted he does say that it is three because three of the light green (named as 1) fit in. It is this same relationship which the second child now needs to label one third(?). After giving her time to think, the others prompt her by asking how many of the whites fit it and placing whites near the rod. This is enough to lead her to say “one third”. I recognize that it will be key when introducing fractions to the younger children for them to have an awareness that 3 means three of whatever is one. This awareness is allowing them to create names by comparison. The red is named as two thirds “because two of the third fit into it”. The green is named as three thirds. This is a start, but there seems to be a danger of them naming rods in succession using their length as measured by the white – pink as four thirds, yellow as five thirds, etc. But this will involve only limited comparison, so I try to “give the effort another twist and make another demand on the children” by setting out an order of rods to be named for this next activity p.7 (Goutard 1964,2017).
Figure 2.4: CI2B
Clip Cl1B demonstrates another feature of the empirical phase. Children are free to be creative in their labeling with the result of more complexity emerging. On the white board the blue has been named as 1, so by having to name light green, they call it one third of 1, then dark green as two thirds of 1. White is named as one ninth, but red instead of being named as two ninths has been named as one third of two thirds of 1. Light green is then renamed as half of two thirds of one. By generating fraction names through making comparisons, the possibility of addition, multiplication and subtraction of fractions presents itself early and is accepted by the children as producing logical names. I notice that children will write an operation on a fraction – e.g. 2 x 1/3 before they will name it as 2/3. They are using something they are familiar with, they do not ‘invent’ the condensed name 2/3 – why should they when they have a perfectly useful and familiar alternative?
Figure 2.5: CI2C
Clip Cl1C. Children seem to like complexity which leads naturally to equivalent fraction names. I notice they often add a new twist to the game themselves to satisfy their sense of fun at devising increasingly involved names. The first child starts to name the tan rod as eight fifths, but a ‘more interesting name” is suggested. He then responds with ‘four fifths plus four fifths’, this uses the labeling of the act of placing rods end to end as ‘plus’ to naturally lead to addition of fractions. He now needs to be encouraged to leave the name eight fifths. The children are comfortable with the idea of a fraction having two or more names. An opportunity has occurred for moving towards systematisation. These fraction names could be saved and studied later when more similar examples have been gathered.
Figure 2.6: CI2D
Clip Cl1D. This activity takes place several weeks after the previous videos. I am wondering how I will know when the empirical phase is no longer valuable. Goutard warns “Nevertheless it would not be wise to shorten this phase of the investigation and throw oneself straight into a systematisation of facts, as the danger of drying up the minds of the pupils exists. This phase is the fountain-head of wealth, facility and technical knowledge needed for the future.” p.7 (Goutard 1964,2017). So in this clip I am looking to see if there is anything ‘fresh’ in their comparisons; if this empirical work is still worthwhile. I notice that they are anticipating new names more quickly now, based on previous names (one child says says ‘you’ve given away another name’ as if it is obvious) but also that one child’s reasons for naming refers to complements to one whole – she justifies the name 6/10 by referring to adding 4/10 or 2/5 to make a whole, then 7/10 as needing 3/10 more. This seems to justify fostering this creative approach as the children meet the need for handling fractions in different ways. Perhaps activities at the empirical phase which generate certain types of writings could be used in a more focused way to intentionally lead to systematisation.
“Since mathematics only exists in the mind and by the mind, the use of the material cannot be everything. ……True, notation rests upon the experience acquired through the manipulation of the rods but it can only come on its own when the mind leaves the material and masters the significance of the manipulations. Between the perceptive exploration of the material and notation, the important phase of the conscious elaboration of the experience takes place.” p.35 (Goutard 1964,2017)
So, I need to give the children the opportunity to become freed from memory or the material itself. At this point, the use of the rods may be ‘keeping the children at too empirical a mode of thought’. Goutard suggests that children should be given the chance to write their own mathematics daily, and is adamant that this should take place when the rods have been put away, hence allowing conscious elaboration of the experience to take place.
(see Samples of the children’s writing Section 2.2.3)
This is useful for assessing individual progress, but by its very nature, this leads to children working at very different levels of thought at one time, so the challenge remains to plan activities allowing for this variety but also moving generally towards systematisation and mastery of structures. In the following activities devised for years 3 and 4, I am aiming to do this. I want to continue this freedom of expression and creativity but I also want the children to work at the level of structures, by seeing patterns and numerical relationships, which can still be verified by comparison to other lengths already named. I do not want them to use a ‘rule’ without recourse to their own logic. This may point to a danger of imposing a ‘mastery of structure’ upon children who have not discovered it for themselves therefore cannot verify it against their own understanding.
Class 3 (Years 4 and 5) Video sequence “If that’s…. then this would be..” (Cl3A to C)
Figure 2.7: Cl3A
In clip C13A children have been given 2 orange rods to represent the 1 litre mark on a jug. They are asked to label other possible divisions through rod comparisons. First the white is named as one fortieth of two litres, red is then named as one twentieth of two litres, and pink as one tenth of 2 litres. One child sees the name one twentieth as meaning that it fits in 20 times, whereas another notices that the denominator has halved as the rod size has doubled. Naming the pink after the red allows her to extend her theory. The two justifications for the fraction name are presented simultaneously in the group. This is worth preserving in activities.
Figure 2.8: CI3B
In clip Cl3B Three children discuss the name for orange and yellow placed end-to- end. One child has named it 1.5, but by reminding her that 10 oranges is 1, another shows that it must be 0.15 meaning one tenth and 5 hundredths. The children are able to confirm equivalent fraction and decimal names by referring back to previous comparisons.
Figure 2.9: Cl3C
In clip Cl3C the children become aware that a fraction can have more than one name, I ask them to pull out names for one fraction and compare them. They quickly identify equivalent fractions where numerator and denominator are doubled and some suggest relationships of multiplying or dividing by other numbers. They continue a naming activity, but with this new awareness fresh in their minds. This group has decided to draw a vertical line between equivalent fractions to check if they fit this rule. Importantly, the names are still generated by comparison.
These activities do not always achieve what I was hoping for. Watching the clips shows that children are often only following one method of comparison at a time – even in groups they will agree another child’s name and reason, but will return to their own route for establishing a name when it is their turn, so not really taking on another view point. A new task is needed requiring the children to establish the logical name by more than one comparison simultaneously to see how these operations are related to one another.
And also, it must be, because (Cl3D to Gb)
The activity generating equivalent names by empirical thought is now much shorter, with the children now being asked to find a ‘route’ from one name to another. They choose a name and explain why it can be substituted by another name. I believe this sequence illustrates an overlap between empirical phase and systematisation/mastery of structure.
Figure 2.10: Cl3D
In clip C13D Two children suggest names for rods using fractions, decimals and percentages simultaneously, reasoning with reference to previous rods. I think they are discovering new connections between names as they talk.
Figure 2.11: Cl3E
In clip Cl3E of the same activity, I notice how much the children’s confidence with reasoning has grown. They have become used to explaining their thinking aloud.
Figure 2.12: Cl3F
I found clip Cl3F interesting because one child raises her own question about 4/4. Her partner explains it to her but she doesn’t accept his explanation without finding her own ‘route’. This strikes me as exactly the kind of discussion I want to encourage, where children can genuinely learn from each other.
Figure 2.13: Cl3Ga
The group in clip Cl3Ga have been generating equivalent names for fractions by comparison with other named rods, then checking that they fit their new theory that the numerator and denominator can be multiplied/divided by the same number to generate an equivalent fraction name. This seems to be close to mastery of structure, but to be sure I set out four fraction names and ask how they are related.
Figure 2.14: Cl3Gb
I think in clip Cl3Gb the child seems to have achieved some mastery of the situation. One girl is looking for a fraction they ‘go back to’. It seems that she is able to see that “every element or group of elements is seen to potentially contain the infinite set of which it is part, as soon as the dynamic links between the elements have been noticed.” (p.18 (Goutard 1964,2017) describing mastery.)
Conclusions (Part 1)
- The value of the empirical phase
In these activities children use what they already know and apply it to a new operation. Goutard says children should be given what little they need and allowed to discover the rest. With relation to fractions, the little, seemed to be for example, the condensed 3⁄4 to replace 1⁄4 + 1⁄4 + 1⁄4. They were able to use adding, subtracting and multiplying fractions, to write mixed number and ‘top-heavy’ fractions and discover equivalent fraction names with no previous teaching of these areas. The value is that there is always an internal logic of the process to verify the name without needing a teacher or rule. Children need time to make their own discoveries, to see their own links and to build up a series of links which can later be drawn as evidence to reinforce understanding of structure. This stage is time-consuming, but necesary for deeper understanding. This explains to me why Goutard felt it was so important to start working on the four operations simultaneously from children’s very first encounters with mathematics.
- How to move children towards systematisation and mastery of structure By asking for names of fractions to be generated in a specific order or with some other limitation on naming, I aimed to put the children in a situation where structures became more apparent. Some children will just ‘see’ the structure, but the overlap with empiricism is important for them to remember how they arrived at their understanding so that they can re-create it at any time independently.
Explore Part 2 and watch the videos about the signs that an attempt at systematisation is being made and what triggers these attempts.
Part 2 discussion
What are the signs that an attempt at systematisation is being made and what triggers these attempts?
At this point in my research I re-read Goutard’s chapter ‘The Danger of Empiricism’ and remembered that she was writing about the very youngest children. Above, I have illustrated the empirical phase with Years 2 and 3 and moving towards systematisation and mastery of structure with Years 4 and 5, but Goutard does not relate these phases to age and suggests that children can be at different stages with different operations at any one time. “The three phases that we have found in the process of structuration do not correspond to any chronological stages in the development of intelligence. It would be unhelpful to associate one age level with empirical investigation, another more advanced to the period of systematisation and so on…Most ten year old children have only fragmentary and empirical knowledge of mathematics because they have never been given a chance to explore it adequately. In contrast, children of 6 can, with a proper education, master structures. …There will be some overlapping of the three pedagogical phases …they may be at a more advanced stage with respect to additions and subtractions and be at the empirical stage only with respect to fractions, which form a more complicated set” p.28 (Goutard 1964,2017).
So I began to look for the three phases of working with Reception and Year 1 children, keen not to miss opportunities for moving beyond empiricism.
Video clips Year 1, term 1. Writing addition statements: “I know it because” (Cl1A to C)
Again, I needed an activity in which the children articulate their ideas to others allowing me to hear their reasoning. In this sequence of clips the Year 1 children are writing their own statements using the knowledge they have gained about the rods.
Figure 2.15: Cl1A
In clip Cl1A the child writes d = g + g on the interactive whiteboard then sets out rods to show her group what her writing means. The rods are used to explain her thinking. This seems to be empirical thought, but its value is in exploring use of the mathematical signs.
Figure 2.16: Cl1B
Again, clip Cl1B seems to demonstrate the same value of this empirical activity for learning to use signs. The child writes y = g + r. When she reads this to the group she says ‘yellow plus green equals red’. When she asks the group if they agree they say ‘no’. She then tells them that she means ‘yellow on its own and green and red together’. She places the rods in this arrangement. When she reads her statement again she reads the = as ‘plus’ but quickly corrects herself. This shows she is clear about what she wants to say, but it not yet sure of how the signs relate to it. I notice that she writes y = g +, stops to look up at the pictures of the rods, then nods before writing ‘r’. She seems to be gathering empirical evidence.
Figure 2.17: CI1C
In clip Cl1C the child seems to have mastered the signs and be ready for more systematic work. He writes confidently, saying aloud what he means and what the symbols communicate. He asks the others if they agree showing that his written mathematics is a form of communication. He drags the rods into place in the same order he has written them, but I wonder if it is necessary for him to illustrate it with the rods; couldn’t he use other reasoning to verify his statement and would this take him beyond the empirical phase? Are the rods keeping him too long at the empirical phase?
“..answers are not what matters most and children are capable of finding them mentally once teachers learn not to ascribe much importance to them but to watch the dynamics which serve as their basis instead” p.3 (Goutard 1964,2017})
This is what I am trying to find out from the child in the next clip – the dynamics which serves as the basis of his statement.
Figure 2.18: Cl1D
Although he uses the rods to explain how his signs should be interpreted, they do nothing to verify his statement about equivalent length because they are not accurately drawn or easy enough to position exactly. In terms of his understanding this doesn’t seem to matter. I believe he knows enough about the relationship between blue and orange and between white and red to justify his writing. Notice that he smiles confidently and is not concerned that the rods don’t fit – he just overlaps them slightly! This, along with his attempts at explaining his reasoning, seems to suggest that he is ready for more challenges which will take him beyond empirical thought.
Conclusions
The empirical phase is useful in confirming the child’s intentions when using a mathematical sign, ensuring that we share an understanding of the situation it signifies. It is useful for the children to gather information which they can organise later, but there seems to be a point at which the child no longer needs the rods to prove their statements and at this point they need to be put in situations where they can explain their reasoning in other ways. The following clips show activities aimed at doing this. (Cl1 E to H)
Figure 2.19: CI1E
Children are trying to find different ways of matching the length of the orange rod with other rods. I believe this work is at the empirical phase but already shows some evidence of systematisation. Substitution and the commutative nature of addition have been used in successive rows of rods showing that some level of awareness is present.
Figure 2.20: Cl1F
In clip Cl1F, the children are setting out partitions of the yellow rod and have been asked ‘have you got them all?’ One child begins a more systematic approach by grouping partitions by colour.
Figure 2.21: Cl1G:Pattern for the dark green (Year 1) [d]
Again in response to the question ‘do you think you have got them all?’ groups set about organising rods into different groups and explain their flow of ideas.
Figure 2.22: Cl1H
Following Goutard’s advice to move away from the rods in order to achieve mastery of structures, I try asking two children to tell me in clip Cl1H how many ways they could make yellow. Rather than remembering partitions, they seem to be reasoning about them using the commutative law and substitution as they go along: One suggests it could be done ‘the other way round’, another mentally substitutes red for two whites.
Conclusions (Part2)
In these examples it seems to be the questions ‘have you got them all?”, ‘how can you be sure?’ and ‘is there a way of checking?’ that prompt systematic work. Ideally, I would like these questions to come from the children, but this will require careful planning.
In an attempt to hear children articulate their understanding of an operation, I ask the children to write what they know about different rods (without the rods present), hoping that they will reveal any knowledge of structure they have gained. (Cl1I to Md)
Figure 2.23: Cl1I
In Cl1I the child uses her knowledge of subtraction as the inverse of addition to explain her statement.
Figure 2.24: Cl1J
In Cl1J she goes on to use substitution to explain her second statement – that orange can be replaced by p + d
Figure 2.25: Cl1K
The child in clip Cl1K uses substitution to cancel out letters in his equation, then is left with a commutative statement which he feels to too obvious to need explanation!
Using one of Goutard’s activities, I then ask the children to re-write a statement, altering only one part, again hoping to see what structures they are aware of.
Figure 2.26: CI1L
In clip Cl1L children take turns to suggest equivalent expressions. They seem to show knowledge of addition as commutative and use substitution, but it would have been more useful to ask them to explain their statement aloud to hear their reasoning.
In this final sequence of clips I present the children with a series of equations, typical of those they had written for themselves in earlier lessons. Each equation is a transformation of the previous one. I was interested to see if the children’s understanding of structure was sufficient to allow them to follow someone else’s transformations. I try various approaches to encourage them to reason individually, with a partner and as a group. Ma, b, c, d
Figure 2.27: CI1Ma
Figure 2.28: CI1Mb:Substitution and Transformation (Year 1) p + r + r = o - r
Figure 2.29: CI1Mc:Reasoning about substitution and transformation p + r + w + w = o - (w + w)
Figure 2.30: CI1Md:Substitution and Transformation (Year 1) 4r = (o + r) - p
Conclusion
At this point believe I have seen that even the youngest children can move away from empirical thought if given the opportunity. This shift seems to take place when the rods are not present and children are in the position of transforming a statement, either orally or in writing, using what they know to be possible with an operation. This seems to explain the importance of independent writing by the children, not as a way of showing what they know, but as an opportunity to try out the structures they are beginning to see.
Next steps
At this point in my research, I have attempted to interpret Goutard’s theory into classroom teaching and have seen what I believe to be examples of her three phases of working, even with the youngest children. I am beginning to understand her concerns that children are limited by too empirical a mode of thought, having seen evidence of systematisation and even mastery in some children after a very short time working with a new operation. Even after such in-depth study of the texts, I still have much more to learn, but I believe that it is only through classroom-based research that I can find out more about young children’s remarkable abilities as mathematicians.
![Grade 1 Student aged 6, after four months at school. page 177 [@xie2018]](images/goutard.png)